Draw a triangle, any triangle (although it may be best to start with an acute triangle). I will instruct you to mark nine special points on your picture.
1) Mark the midpoints of each side (3 points). See Figure 1.
2) Drop an altitude from each vertex to the opposite side, and mark the points where the altitudes intersect the opposite side. (If the triangle is obtuse, an altitude will be outside the triangle, so extend the opposite side until it intersects.) See Figure 2.
3) Notice that the altitudes intersect at a common point. Mark the midpoint between each vertex and this common point. See Figure 3.
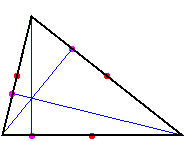
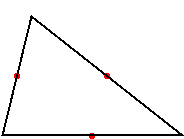
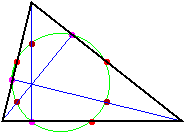
No matter what triangle you start with, these nine points all lie on a perfect circle!
Presentation Suggestions:
Do an example on the board as you give these instructions, and have students draw their own triangles. Be sure to start with an acute triangle first, because the construction with an obtuse triangle can be confusing. Also, try not to draw a triangle that is equilateral, isosceles, or right, because in those degenerate cases, some of the points will overlap. Practice before you do one in class!
The Math Behind the Fact:
Even simple geometry still has some surprises in store! This result was known by Euler in 1765, but rediscovered by Feuerbach in 1822. The resulting circle is known as the Feuerbach circle or nine point circle. The reference contains a proof.
How to Cite this Page:
Su, Francis E., et al. “Nine Point Circle.” Math Fun Facts. <http://www.math.hmc.edu/funfacts>.
References:
H. Dorrie, 100 Great Problems of Elementary Mathematics, Dover, 1965, pp.142-144.
Fun Fact suggested by:
Francis Su

