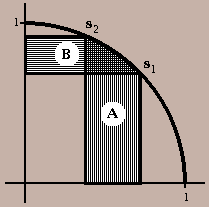
Consider a unit circle, and any arc S on the unit circle in the first quadrant. No matter where S is placed, the area between S and the x-axis plus the area between S and y-axis is constant! Moreover, that constant is equal to the length of S:
A + B = s2 – s1.
In Figure 1, note that regions A and B overlap; in that portion the area is counted twice. The quantity (s2 – s1) represents the length of S along the arc from s2 to s1.
Presentation Suggestions:
Draw a couple of pictures with arcs of the same length in different positions. Perhaps assign the computation of the areas as a fun homework exercise.
The Math Behind the Fact:
Use calculus, or sector-triangle formulas from geometry, to compute the corresponding areas.
How to Cite this Page:
Su, Francis E., et al. “Arclength Surprise.” Math Fun Facts. <http://www.math.hmc.edu/funfacts>.
References:
Putnam examination, 1998, in The William Lowell Putnam Mathematical Competition 1985-2000.
Fun Fact suggested by:
Francis Su

