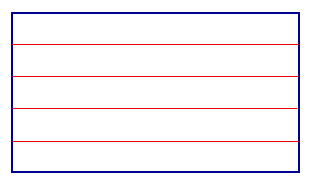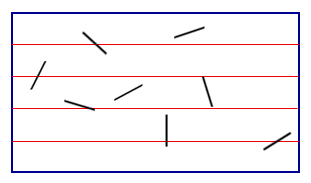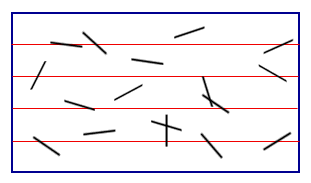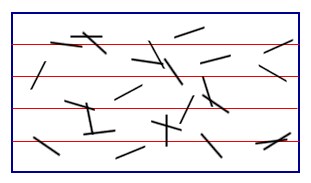
A plane is ruled with parallel lines 1 cm apart. A needle of length 1 cm is dropped randomly on the plane. What is the probability that the needle will be lying across one of the lines?
Answer: 2/Pi.
This gives an interesting way to calculate Pi! If you throw down a large number of needles, the fraction of needles which lie across a line will get closer to 2/Pi the more needles that you throw. So, you can just throw down needles and count them to get an estimate for Pi!
Presentation Suggestions:
Draw a picture and a few “random” needles. As a challenge, ask students to prove this formula using calculus, and assuming that needle centers and needle angles are uniformly distributed.
The Math Behind the Fact:
This method of calculating Pi is very slow. There are faster formulas, see pi formula. However, the idea of using a probabilistic means to get answers like this is very powerful, and is the basis of something called the Monte Carlo method in probability theory.
How to Cite this Page:
Su, Francis E., et al. “Buffon Needle Problem.” Math Fun Facts. <http://www.math.hmc.edu/funfacts>.
Fun Fact suggested by:
Francis Su

