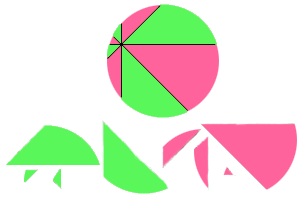
Take a pizza and pick an arbitrary point in it. Suppose you cut the pizza into 8 slices by cutting at 45 degree angles through that point, and color the alternate pieces red and green.
Surprising theorem: the total area of the red slices and the total area of the green slices will always be the same!
In fact, this theorem is true if the number of slices is any multiple of 4 except for 4, and the slices are cut by using equal angles through a fixed arbitrary point in the pizza.
Alternatively, if instead of equal angles, you use equal-length arcs on the circumference and slice from a fixed arbitrary point in the pizza, the conclusion still holds if the number of slices is even and greater than 2.
Presentation Suggestions:
Draw a few pictures to illustrate some special cases.
The Math Behind the Fact:
The theorem can be proved by using calculus and polar coordinates. The reference gives background and generalizations.
How to Cite this Page:
Su, Francis E., et al. “Pizza Slices.” Math Fun Facts. <http://www.math.hmc.edu/funfacts>.
References:
J. Konhauser, D. Velleman, S. Wagon, Which way did the bicycle go?, pp. 20, 117-122.
Fun Fact suggested by:
Francis Su

